- Related video from YouTube
- Probability Basics
- Probability Distributions for Dice
- Optimal Strategies
- Advanced Techniques
- Conclusion
- FAQs
Dice Probability: Strategies to Win Games
Understanding dice probability is crucial for making informed decisions and gaining an edge in dice games. Here are the key strategies:
Probability Basics
- Probability quantifies the likelihood of an event occurring
- Each dice roll is an independent event, not affected by previous rolls
- Expected value helps evaluate potential payoffs and risks for decision-making
Probability Distributions for Dice
| Dice Rolls | Possible Outcomes |
|---|---|
| 1 die | 6 |
| 2 dice | 36 |
| 3 dice | 216 |
Optimal Strategies
- Focus on bets with higher expected value and lower risk
- Use probability to identify favorable betting opportunities
- Manage risks by understanding potential outcomes and their probabilities
Advanced Techniques
- Maximize expected value by weighing potential benefits against risks
- Use simulations and programs to analyze complex games and optimize strategies
- Factor in your personal risk preferences when making decisions
To improve your chances of winning dice games, understand probability theory, analyze outcome distributions, and develop optimal strategies based on expected value and risk management.
Related video from YouTube
Probability Basics
Understanding Probability
Probability is the mathematical study of how likely an event is to occur. It helps us quantify and analyze the chances of different outcomes. Here are some key concepts:
- Sample Space: All possible outcomes in an experiment or event.
- Event: A specific set of outcomes from the sample space.
- Probability: A number between 0 and 1 that represents the likelihood of an event happening.
The probability of an event is calculated by dividing the number of favorable outcomes by the total number of possible outcomes in the sample space.
Independent Dice Rolls
In dice games, each roll of the dice is an independent event. This means that the outcome of one roll does not affect the outcome of any other roll. The probability of rolling a specific number on a single die remains the same, regardless of previous rolls.
For example, when rolling a standard six-sided die, the probability of rolling a 3 is always 1/6 or approximately 0.167, no matter what was rolled before. This principle of independent events is crucial for calculating probabilities for multiple dice rolls.
Expected Value in Decision-Making
The expected value represents the average or expected outcome of an event or experiment. It is calculated by multiplying each possible outcome by its corresponding probability and then summing those values.
In dice games, the expected value can help players make informed decisions by evaluating the potential payoffs and risks associated with different choices or strategies. By considering the expected value, players can optimize their decisions to maximize their chances of winning or minimizing their losses.
For example, in the game of Craps, players can use the expected value to determine the most advantageous bets to make based on the probabilities of different dice roll outcomes.
| Concept | Description |
|---|---|
| Sample Space | All possible outcomes in an experiment or event |
| Event | A specific set of outcomes from the sample space |
| Probability | A number between 0 and 1 representing the likelihood of an event occurring |
| Independent Dice Rolls | Each roll of the dice is an independent event, not affected by previous rolls |
| Expected Value | The average or expected outcome of an event or experiment, used for decision-making |
Probability Distributions for Dice
Single Die Outcomes
When rolling a single six-sided die, there are six possible outcomes: 1, 2, 3, 4, 5, and 6. Each outcome has an equal chance of occurring, which is 1/6 or about 0.167.
| Outcome | Probability |
|---|---|
| 1 | 1/6 |
| 2 | 1/6 |
| 3 | 1/6 |
| 4 | 1/6 |
| 5 | 1/6 |
| 6 | 1/6 |
Two Dice Outcomes
When rolling two six-sided dice, there are 36 possible outcomes. The probability of each outcome is calculated by multiplying the probabilities of each die. For example, the probability of rolling a 3 on the first die and a 4 on the second die is (1/6) × (1/6) = 1/36.
Here's a table showing the probability distribution for rolling two six-sided dice:
| Outcome | Probability |
|---|---|
| 2 | 1/36 |
| 3 | 2/36 |
| 4 | 3/36 |
| 5 | 4/36 |
| 6 | 5/36 |
| 7 | 6/36 |
| 8 | 5/36 |
| 9 | 4/36 |
| 10 | 3/36 |
| 11 | 2/36 |
| 12 | 1/36 |
Three Dice and More
As the number of dice increases, the probability distribution becomes more complex. For three dice, there are 216 possible outcomes, and for four dice, there are 1,296 possible outcomes. The probability of each outcome is calculated using the multiplication principle, but it becomes impractical to list all possible outcomes.
Instead, we can use probability distributions to model the behavior of multiple dice rolls. For example, the binomial distribution can be used to model the number of successes (e.g., rolling a 6) in a fixed number of trials (e.g., rolling three dice).
Calculating Specific Probabilities
To calculate the probability of a specific event, such as rolling a sum of 7 with two dice, follow these steps:
- Identify the possible outcomes that satisfy the event (e.g., rolling a 3 and a 4, or rolling a 2 and a 5).
- Calculate the probability of each outcome using the multiplication principle.
- Add up the probabilities of each outcome to get the total probability of the event.
For example, to calculate the probability of rolling a sum of 7 with two dice, we can identify the possible outcomes as (3, 4), (4, 3), (2, 5), and (5, 2). The probability of each outcome is (1/6) × (1/6) = 1/36. Since there are four possible outcomes, the total probability of rolling a sum of 7 is 4/36 = 1/9.
sbb-itb-9dfdbdd
Optimal Strategies
What is an Optimal Strategy?
An optimal strategy in dice games is the best approach to increase your chances of winning while reducing risks. It involves making smart decisions based on probability, risk management, and understanding the game rules. By using an optimal strategy, you can improve your odds and minimize losses.
Managing Risks
Risk management is crucial for optimal strategy in dice games. It means identifying and dealing with potential risks, such as losing a bet or not achieving the desired outcome. By understanding and managing risks, you can make better decisions and optimize your strategy.
Using Probability
Probability plays a vital role in optimal strategy for dice games. By understanding the probability of different outcomes, you can make informed decisions about which bets to place, when to hold, and when to roll again. For example, in Craps, knowing the probability of rolling certain numbers can help you decide which bets to make and when to take odds.
Example: Craps
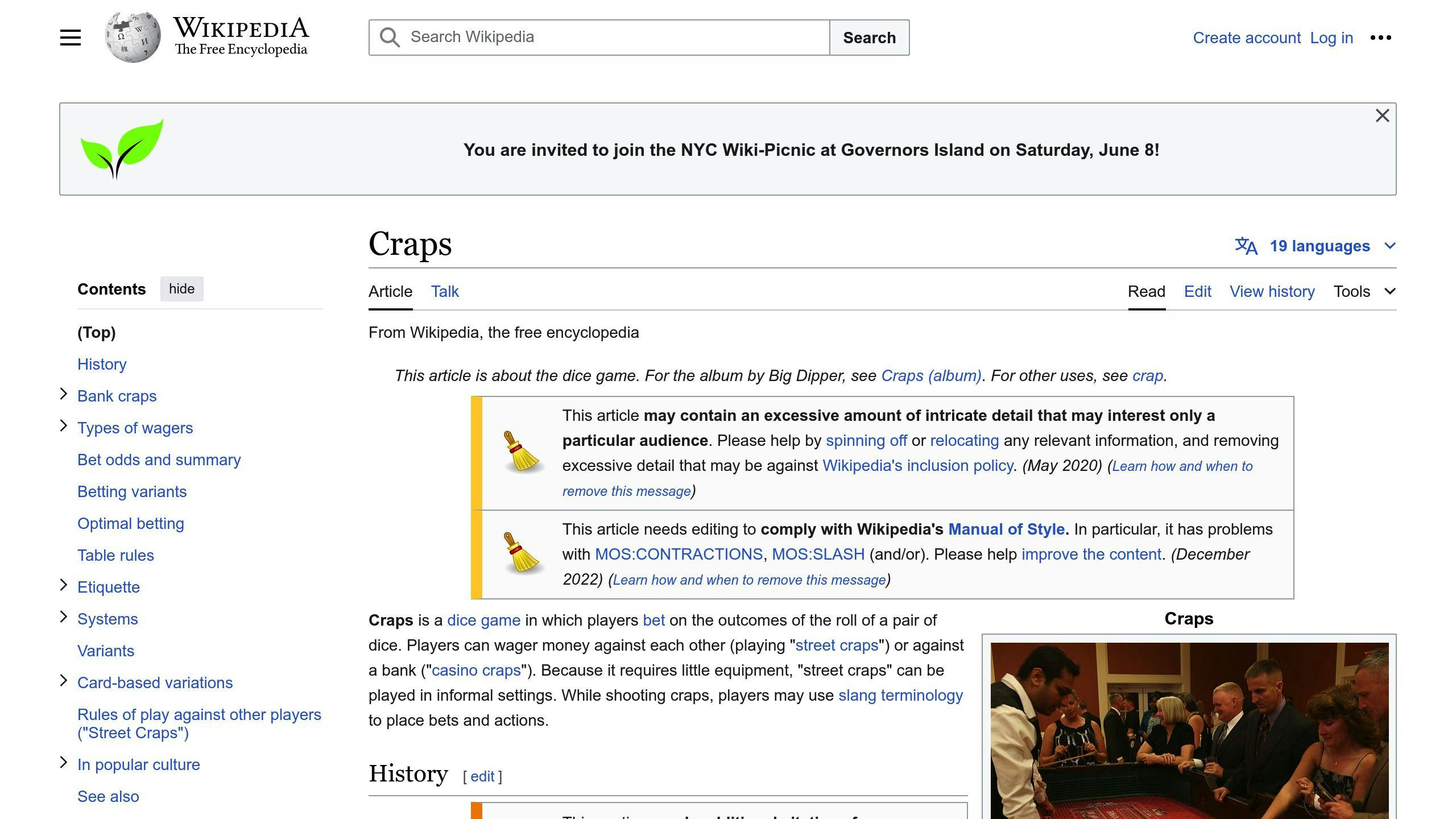
In Craps, an optimal strategy is to focus on the "pass line" bet, which has a relatively low house edge. By understanding the probability of rolling certain numbers, you can decide when to take odds and when to hedge your bets. You can also use probability to identify favorable betting opportunities, such as placing bets on the "don't pass" line when the shooter is likely to roll a 7.
Example: Pig

Pig is a simple dice game where you roll a die and try to score points. An optimal strategy in Pig is to roll again when your score is low, as the probability of rolling a higher number is relatively high. By understanding the probability of rolling certain numbers, you can decide when to hold and when to roll again. You can also use probability to identify favorable scoring opportunities, such as rolling again when your score is close to a multiple of 10.
| Game | Optimal Strategy |
|---|---|
| Craps | Focus on "pass line" bet, take odds, hedge bets, identify favorable betting opportunities |
| Pig | Roll again when score is low, hold when score is high, identify favorable scoring opportunities |
Advanced Techniques
Maximizing Expected Value
When playing dice games, it's crucial to consider the expected value of each possible outcome. The expected value is the average return or profit you can expect from a particular action or decision. To maximize your chances of winning, you need to weigh the potential benefits against the potential risks.
For example, in Craps, the expected value of placing a "pass line" bet is higher than placing a "don't pass" bet because the probability of winning is higher. By understanding the expected value of each bet, you can make informed decisions about which bets to place and when to take odds.
Using Simulations and Programs
Simulations and computer programs can be powerful tools for analyzing and developing strategies for complex dice games. By running simulations, you can test different scenarios, identify patterns, and optimize your strategy.
For instance, you can use a simulation to determine the best betting strategy in Craps based on the probability of rolling certain numbers. You can also use programs to analyze large datasets and identify trends that can inform your decision-making.
Factoring in Risk Preferences
Your personal tolerance for risk can significantly impact your decision-making in dice games. To factor in risk preferences, you need to consider how much risk you're willing to take and adjust your strategy accordingly.
| Risk Preference | Strategy |
|---|---|
| Risk-Averse | Stick with conservative bets and avoid taking risks |
| Risk-Seeking | Take bold bets and push the limits |
Conclusion
Key Points
In this guide, we covered the basics of dice probability and strategies to boost your chances of winning dice games. We explored:
- Probability theory
- Independent dice rolls
- Expected value
- Optimal strategies for informed decisions
We also discussed advanced techniques like maximizing expected value, using simulations and programs, and considering your risk preferences.
Final Thoughts
Grasping dice probability is key to making smart decisions and enjoying dice games more. By applying the strategies and techniques from this guide, you can increase your odds of winning. Remember, it's not just about luck - it's about using the odds to your advantage.
Putting It into Practice
Now that you've learned these strategies, it's time to put them to use. Start applying the concepts to your favorite dice games and see how they improve your chances. Don't be afraid to experiment with new strategies, but always keep probability and risk management in mind when making decisions. With practice, you'll become a master of dice probability and take your gaming to new heights.
Tips for Success
| Tip | Description |
|---|---|
| Study Probability | Understand probability theory and how it applies to dice games. |
| Analyze Outcomes | Identify all possible outcomes and their probabilities. |
| Manage Risks | Consider potential risks and adjust your strategy accordingly. |
| Use Expected Value | Calculate expected value to make informed betting decisions. |
| Practice | Keep playing and refining your strategies based on experience. |
FAQs
How do you predict the probability of dice rolls?
To predict the probability of dice rolls, you need to understand the chances of getting a specific number or combination. Here's how it works:
- Identify the desired outcome (the number or combination you want to roll).
- Determine the total possible outcomes (all the possible rolls).
- Calculate the probability using this formula:
Probability = Number of desired outcomes / Total possible outcomes
For example, let's find the probability of rolling a 6 on a single six-sided die:
- Desired outcome: Rolling a 6 (1 possibility)
- Total possible outcomes: 6 (rolling 1, 2, 3, 4, 5, or 6)
- Probability = 1 / 6 = 0.17 (or about 17%)
When rolling multiple dice, the calculation gets more complex. You need to multiply the possibilities for each die. For two six-sided dice:
- Total possible outcomes: 6 x 6 = 36
To find the probability of rolling a specific combination, like a 7 with two dice:
- Identify the desired outcomes: (1, 6), (2, 5), (3, 4), (4, 3), (5, 2), (6, 1)
- Count the desired outcomes: 6
- Calculate the probability: 6 / 36 = 0.17 (or about 17%)
| Dice Rolls | Total Possible Outcomes | Example Probability Calculation |
|---|---|---|
| 1 die | 6 | Probability of rolling a 6 = 1 / 6 = 0.17 |
| 2 dice | 36 | Probability of rolling a 7 = 6 / 36 = 0.17 |
| 3 dice | 216 | Probability of rolling three 6s = 1 / 216 = 0.005 |
The more dice you roll, the more complex the probability calculations become. But the basic principle remains the same: identify the desired outcomes, count the total possibilities, and divide to find the probability.